Description
Delve deeper into the fascinating world of number theory with our Advanced Modular Arithmetic course. Designed for students and enthusiasts with a strong mathematical background, this course offers an in-depth exploration of modular arithmetic—a foundational concept in number theory with applications in cryptography, computer science, and various other fields.
Key Topics Covered:
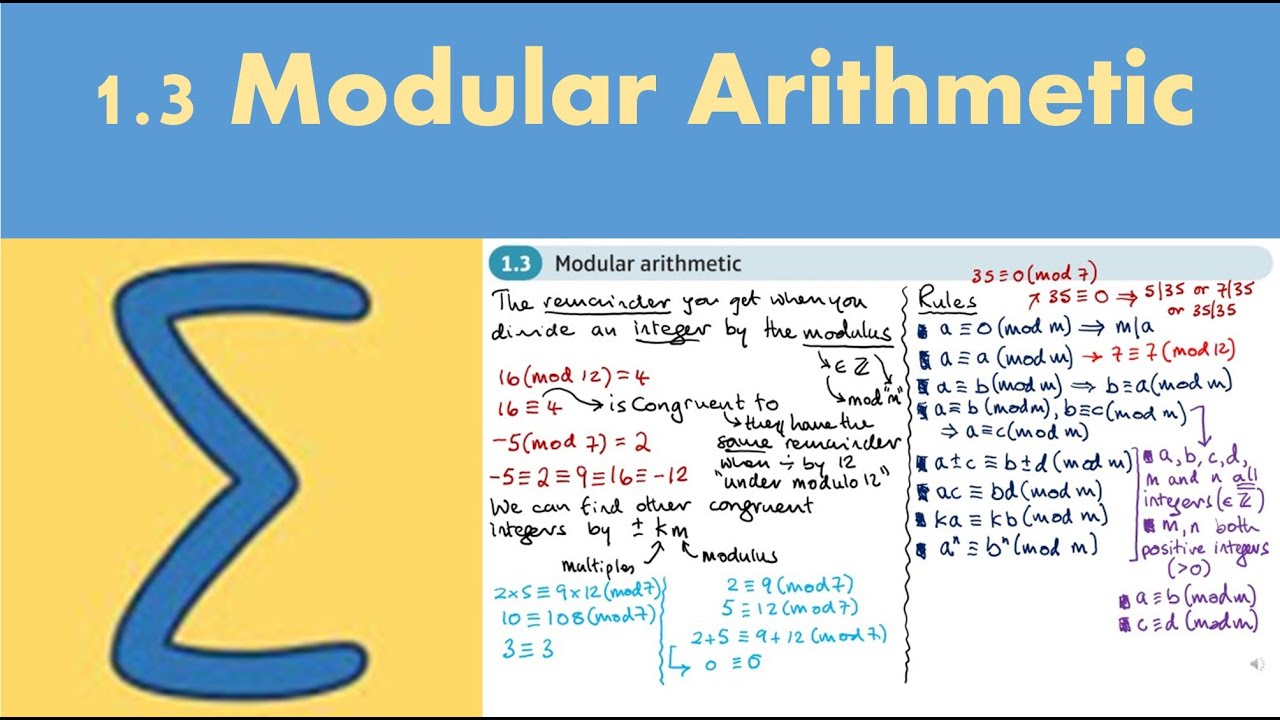
- Introduction to Modular Arithmetic: Review the basic principles of modular arithmetic, including congruence relations and modular operations.
- Arithmetic Properties: Explore arithmetic properties of modular arithmetic, such as addition, subtraction, multiplication, and exponentiation.
- Modular Equations and Systems: Learn techniques for solving modular equations and systems of linear congruences.
- Chinese Remainder Theorem: Understand the Chinese Remainder Theorem and its applications in solving simultaneous congruences.
- Fermat’s Little Theorem and Euler’s Totient Function: Study important results in number theory, including Fermat’s Little Theorem and Euler’s Totient Function.
- Primitive Roots: Investigate primitive roots and their significance in the context of modular arithmetic and discrete logarithm problems.
- Applications of Modular Arithmetic: Explore real-world applications of modular arithmetic, including cryptography, error detection and correction, and pseudorandom number generation.




Risikat –
One of the highlights of this course is its focus on practical applications of modular arithmetic in cryptography. The instructors provide insights into how modular arithmetic forms the foundation of modern cryptographic algorithms, such as RSA encryption. By understanding the underlying principles of modular arithmetic, learners gain a deeper appreciation for the role it plays in securing digital communications and transactions.
Judith –
This course offers challenging and stimulating content that pushes learners to expand their mathematical horizons. The instructors present thought-provoking problems and exercises that encourage exploration and experimentation. The interactive nature of the course fosters a dynamic learning environment where participants can collaborate and exchange ideas, further enhancing their understanding of modular arithmetic at an advanced level.
Eucharia –
The instructors demonstrate a high level of expertise in modular arithmetic, providing in-depth explanations and thorough examples throughout the course. Their passion for the subject shines through in their teaching, inspiring learners to engage with the material on a deeper level. The course not only equips participants with advanced mathematical skills but also fosters critical thinking and problem-solving abilities essential for further exploration in number theory and related fields.
Olubunmi –
“Modular Arithmetic: Advanced Level of Number Theory” offers an advanced and insightful exploration of modular arithmetic. The instructors delve deep into complex mathematical concepts with clarity and precision, making it accessible to advanced learners. The course materials are well-organized, with comprehensive coverage of topics such as congruences, Chinese remainder theorem, and applications of modular arithmetic in cryptography. This course is a must for anyone interested in exploring the fascinating world of number theory at an advanced level.